Distributive Property Definition
To “distribute” means to divide something or give a share or part of something.
So what does distributive property mean in math?
The distributive law of multiplication over basic arithmetic, such as addition and subtraction, is known as the distributive property.
Distributive Property of Multiplication over Addition
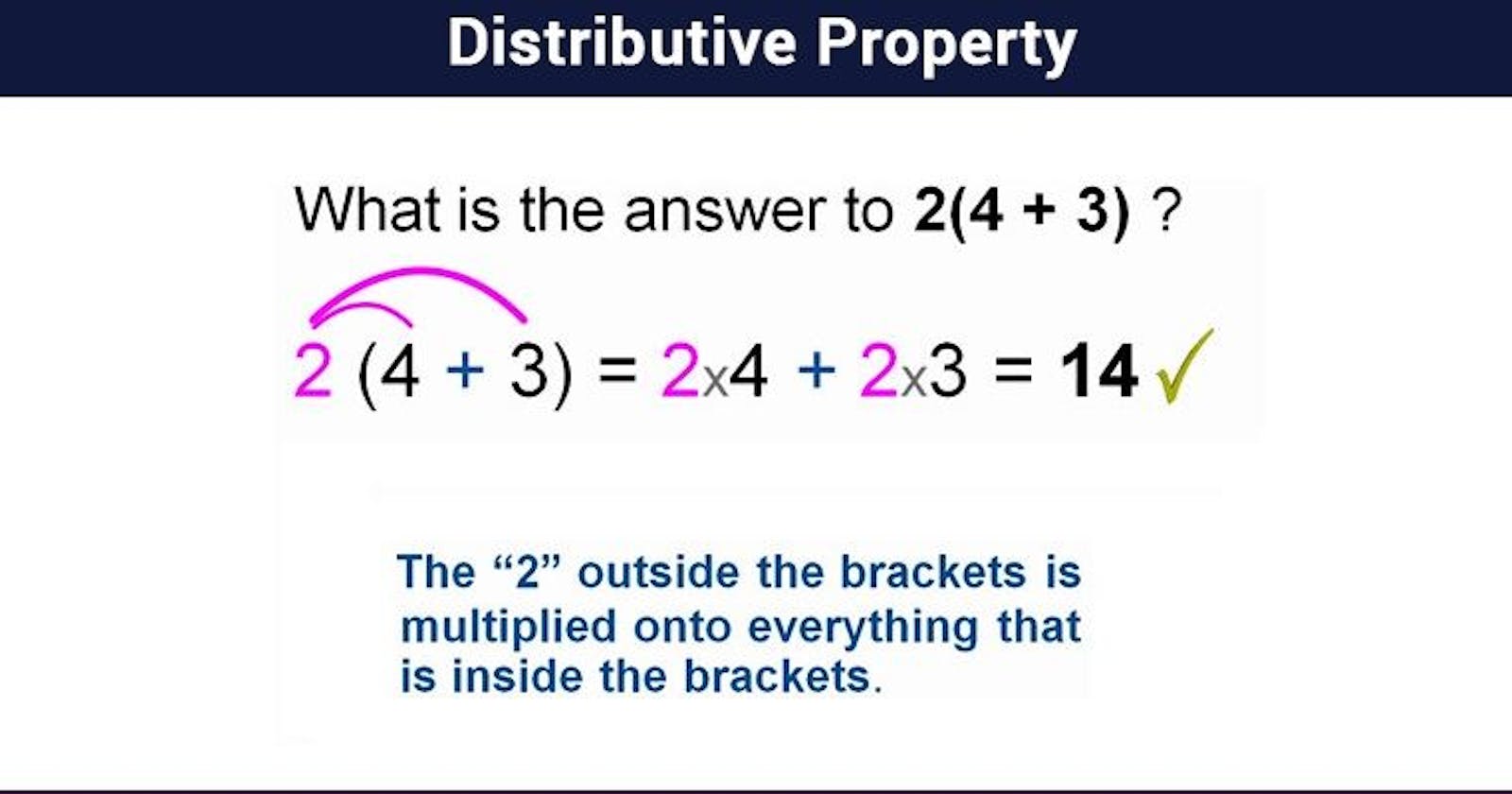
When we have to multiply a number by the sum of two numbers, we use this property of multiplication over addition. Let’s understand how to use the distributive property better with an example:
Example: Solve the expression: 6 (20 + 5) using the distributive property of multiplication over addition.
Let’s use the property to calculate the expression 6 (20 + 5), the number 6 is spread across the two addends. To put it simply, we multiply each addend by 6 and then the products can be added.
6 20 + 6 5 = 120 + 30 = 150
Let’s take another example:
Example: Solve the expression 2 (2 + 4) using the distributive law of multiplication over addition.
Solution: 2 (2 + 4) = 2 2 + 2 4 = 4 + 8 = 12
If we try to solve this expression using the PEMDAS rule, we’ll have to add the numbers in parentheses and then multiply the total by the number outside the parentheses. This implies:
2 (2 + 4) = 2 × 6 = 12
Thus, we get the same result irrespective of the method used.
Fun Facts Even though division is the inverse of multiplication, the distributive law only holds in the case of division, when the dividend is distributed or broken down into partial dividends, which are completely divisible by the divisor.
For instance, using the distributive law for 1326
132 can be broken down as 60 + 60 + 12, thus making division easier.
We cannot break 132 6 as (50 + 50 + 32) 6.
Also, we cannot break the divisor: 132(4+2) will give you the wrong result.
Conclusion
We have understood how distributive property can be used to simplify complex equations and problems. Experience the new way of learning math with turito, which
brings an interactive platform for kids where every concept is turned into a playful session. With interesting sheets, exciting quizzes, and easy-to-understand topics, transform the way your child understands math!
Solved Examples
Example 1: Solve (5 + 7 + 3) 4.
Solution: Using the distributive property of multiplication over addition,
A (B + C) = AB + AC
(5 + 7 + 3) 4 = 5 4 + 7 4 + 3 4 = 20 + 28 + 12 = 60
Or,
(5 + 7 + 3) 4 = 15 4 = 60
Example 2: Solve the following distributive equation −2 (−x − 7).
Solution: Using the distributive property,
A (B – C) = AB – AC
−2 (−x − 7) = (−2)(−x) − (−2)(7) = 2x − (−14) = 2x + 14
Example 3: Which property does the equation 3 (4 − 9) = 3 4 − 3 9 show? Solution: The above equation shows the distributive property of multiplication over subtraction.